Surface-Enhanced Raman Scattering
Raman scattering is a weak effect. If analyte molecules are adsorbed on a plasmonic metal particle surface, the Raman scattering signal can be enormously enhanced. The effect is then referred to as surface-enhanced Raman scattering (SERS). In extreme cases the enhancement is so strong that even single molecule detection becomes possible.
The SERS enhancement mechanism is actually quite complicated. From a theoretical point of view, the total SERS enhancement involves (i) chemical enhancement, (ii) resonance Raman enhancement, (iii) charge-transfer resonance enhancement, and (iv) plasmon resonance enhancement processes [1]. The last contribution is believed to be the largest to the observed SERS signal, and it is often referred to as the electromagnetic (EM) enhancement mechanism.
The EM enhancement
The largest EM enhancement occurs near interacting metal nanoparticles, for example silver or gold. If metal particles are illuminated with light that is in resonance with the particle LSPR frequency, the EM enhancement is particularly strong. The nanoparticle LSPR is generally a function of the particle size, shape and surrounding medium. If there are other nanoparticles nearby, inter-particle coupling effects influence tremendously the LSPR position (“hot spots“).
Consider a simplified electromagnetic enhancement model for SERS in Figure 1. Using the dipolar picture, the incident field can simultaneously excite dipole fields in both particle and molecule. Hence we have two dipole field sources in the system. Since a molecule is in a close proximity to a metal particle, we should expect some kind of coupling between the two dipole fields. It is easier to view this as two processes happening simultaneously.
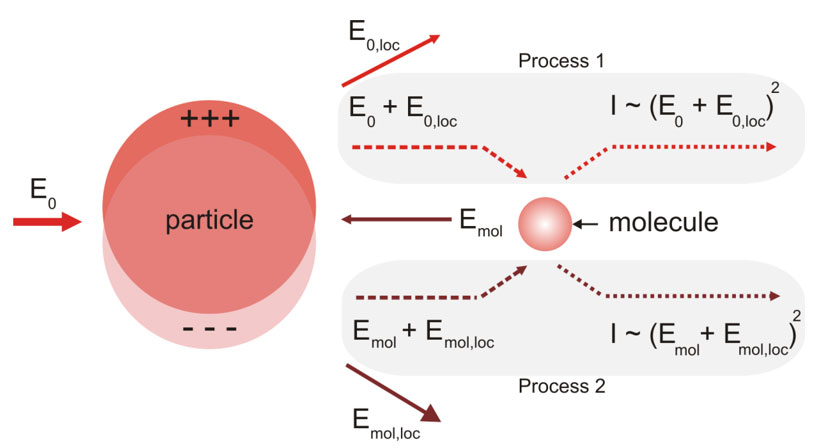
First, we start with a metal particle. The incident electromagnetic field, 
, excites the particle LSP inducing oscillating dipole 
, where 
is the particle polarizability that generally is a tensor. The induced particle polarization generates large local fields 
, i.e. the incident field is enhanced yielding 
. The total field excites the target molecule that emits Raman scattering. The Raman intensity is then 
.
Second, we start with a molecule. The emitted field from a molecule, 
, is also enhanced by a metal particle yielding 
. In other words, the emitted field from the molecule at a certain frequency 
is again enhanced by the particle LSP. The Raman intensity is then 
. Hence, the Raman signal is enhanced by 
in each step.
There is another way to derive the SERS electromagnetic enhancement factor, 
, using the coupled dipole approach.
One can define the dipole moments of a particle and a molecule as follows:
[Eq. 1]
where 
is the incident field, 
and 
are the particle and molecule polarizabilities, respectively. Since the molecule is at the vicinity of the particle surface, we add the particle dipole field ( 
) to the incident field when calculating the dipole moment of the molecule ( 
is a constant). In the same way we add the dipole field from the molecule ( 
) to the incident field when defining the dipole moment for the particle.
The coupled dipole system becomes:
[Eq. 2]
Solving the system yields
[Eq. 3]
Near the particle LSPR 
and the effective particle polarizability 
will dominate the system. The polarizability is modified and is Raman active because there is a contribution from 
. Remembering that the Raman scattering intensity scales as 
, the SERS intensity enhancement becomes
Therefore, the SERS intensity enhancement scales as the 4th power of the ratio between the field in the vicinity of the nanoparticle and the incident field.